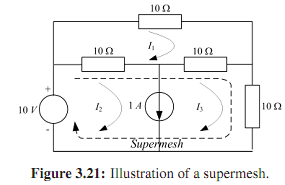
Linearity
-is the property of an element describing a linear relationship between cause and effect.
- The property is a combination of homogeneity(scaling) property and the additivity property.
The homogeneity property requires that if the input (also called the excitation) is multiplied by a constant, then the output (also called the response) is multiplied by the same constant
if v = iR ⇒ kv = kiR
The additivity property requires that the response to a sum of inputs is the sum of the responses to each input applied separately
v1 = i1R, v2 = i2R ⇒ v = (i1 + i2)R = v1 + v2
- We say that a resistor is a linear element because the voltage-current relationship satisfies both the homogeneity and the additivity properties.
- A linear circuit is one whose output is linearly related (or directly proportional) to its input.
Superposition
-The superposition principle states that the voltage across (or current through) an element in a linear circuit is the algebraic sum of the voltages across (or currents through) that element due to each independent source acting alone.
-The superposition principle states that the voltage across (or current through) an element in a linear circuit is the algebraic sum of the voltages across (or currents through) that element due to each independent source acting alone.
To apply the superposition principle, we must keep two things in mind:
1. We consider one independent source at a time while all other independent sources are turned off. This implies that we replace every voltage source by 0 V (or a short circuit), and every current source by 0 A (or an open circuit). This way we obtain a simpler and more manageable circuit
2. Dependent sources are left intact because they are controlled by circuit variables.
1. Turn off all independent sources except one source. Find the output (voltage or current) due to that active source using nodal or mesh analysis
3. Find the total contribution by adding algebraically all the contributions due to the independent sources.
Watch a Video using Superposition principle!
Reflection:
The advantage of superposition is that the circuit can be analyzed with one power source at a time. This will simplify the circuit making the analysis easier. The disadvantage is there is a chance for making an algebraic mistake if we do not keep the same current reference direction each time.